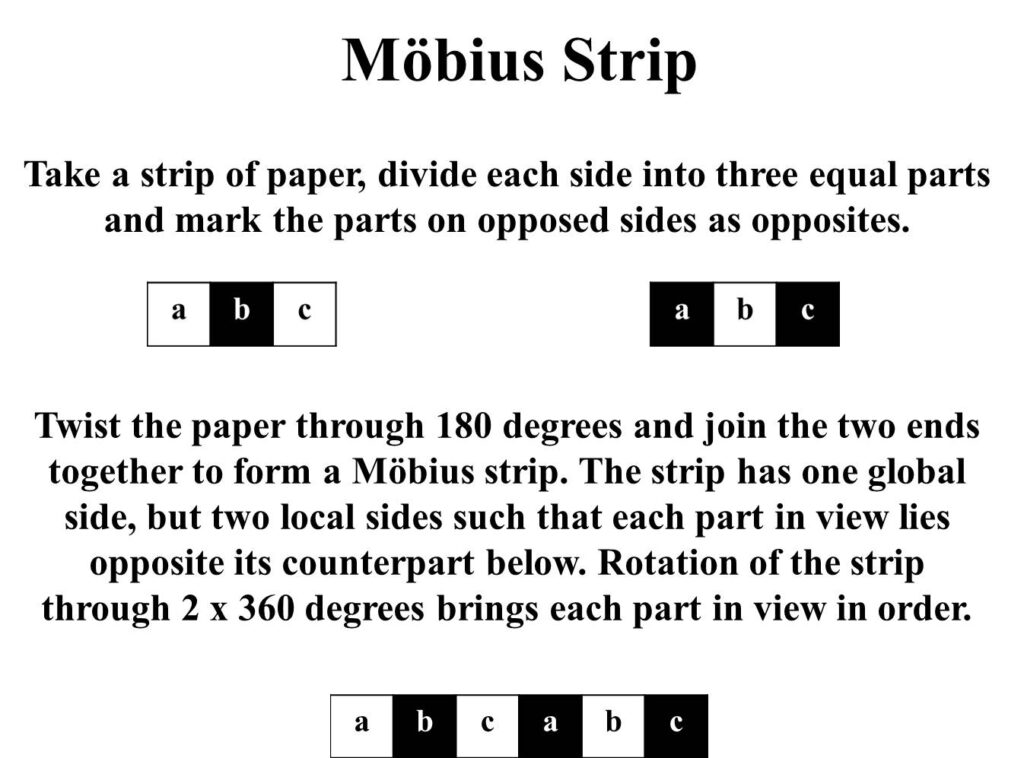
The principles of chiralkine counting can be visualized through the behavior of a Möbius strip, a surface with intriguing properties:
- It has one global side, but this is perceived as two local sides.
- As the strip rotates, parts on one local side come into view sequentially, while their opposite pair members appear on the other side.
Chiralkine Counting and the Möbius Strip
In chiralkine counting, objects and their relationships rotate in a symmetrical cycle, much like a Möbius strip divided into three equal pairs of parts.
- The letters a, b, and c, used in chiralkine counting, can conceptually represent these parts.
- Each part is paired with its opposite, reflecting the symmetry inherent in the counting process.
As the Möbius strip rotates:
- Each part appears in sequence on one side.
- Its opposite pair member takes a corresponding position on the other side.
This mirrors how chiralkine counting maintains symmetry and order as distinctions are drawn and erased cyclically.
Constructing a Möbius Strip
To better visualize this symmetry, a real model can be constructed.
However:
- Instead of letters (which are inherently asymmetric), use primary colors and their opposites to mark the parts.
- Colors better represent the dual, mirror-opposite logic that chiralkine counting embodies.
Conclusion
The Möbius strip offers a powerful analogy for understanding chiralkine counting:
- Both systems involve cyclical progression where opposing relationships are symmetrically intertwined.
- The global unity of the Möbius strip’s surface reflects the inherent symmetry of chiralkine counting, where distinctions continuously cycle through a six-membered rotation.
(Acknowledgement: text drafted by Chat GPT using text provided).